The Science Museum’s Mathematics: The Winton Gallery tells the story of how mathematics has shaped our world. Designed by Zaha Hadid Architects, the new gallery spans 400 years of human ingenuity and brings mathematical history to life through the design and architecture of its displays.
“When I was growing up in Iraq, math was an everyday part of life. My parents instilled in me a passion for discovery, and they never made a distinction between science and creativity. We would play with math problems just as we would play with pens and paper to draw—math was like sketching.” – Zaha Hadid (1950-2016)
There are more than 100 treasures from the Science Museum‘s collection in the Mathematics Gallery that tell powerful stories about how mathematics has shaped, and been shaped by, some of our most fundamental human concerns – from trade and travel, to war and peace, life and death, form and beauty, maps and models, money and computers. How many treasures will you find? What stories will they tell you?
 Positioned at the center of the gallery, IT was built in 1929 to compete in the ‘Safe Aircraft Competition’ for $150,000 in prize money mounted by US philanthropist and industrialist Daniel Guggenheim, and IT is the largest object in the Winton Gallery. IT is famous for its patented slots and flaps which allow the plane to generate lift at slow speeds, thus enabling short take offs and ultimately safer landings. These critical features highlight the successful use of mathematics in the design of the aircraft paving the way to the safe passenger flights that we rely on today.
Positioned at the center of the gallery, IT was built in 1929 to compete in the ‘Safe Aircraft Competition’ for $150,000 in prize money mounted by US philanthropist and industrialist Daniel Guggenheim, and IT is the largest object in the Winton Gallery. IT is famous for its patented slots and flaps which allow the plane to generate lift at slow speeds, thus enabling short take offs and ultimately safer landings. These critical features highlight the successful use of mathematics in the design of the aircraft paving the way to the safe passenger flights that we rely on today. The 42 storeys of this tower completed in 1981 hang over visitors’ heads as they enter. Instead of columns, the floors sit on three huge slabs embedded in the central core, appearing to float. This was only possible by painstaking mathematical analysis. The problem was so complicated the engineers initially ran out of computer space to solve the equations.
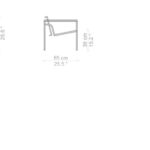
The 42 storeys of this tower completed in 1981 hang over visitors’ heads as they enter. Instead of columns, the floors sit on three huge slabs embedded in the central core, appearing to float. This was only possible by painstaking mathematical analysis. The problem was so complicated the engineers initially ran out of computer space to solve the equations. IT was designed by Charlotte Perriand for Le Corbusier in the late 1920s. Le Corbusier, a pioneer of modern architecture, believed that mathematical rules of proportion, which created harmony with the human form, would lead to beautiful structures, whether chairs or buildings. He was developing ideas first explored by Greek and Roman architects and revived in the Renaissance from the 15th century onwards.
IT was designed by Charlotte Perriand for Le Corbusier in the late 1920s. Le Corbusier, a pioneer of modern architecture, believed that mathematical rules of proportion, which created harmony with the human form, would lead to beautiful structures, whether chairs or buildings. He was developing ideas first explored by Greek and Roman architects and revived in the Renaissance from the 15th century onwards. IT was patented in 1918 by the German engineer Arthur Scherbius (1878-1929), and produced commercially from 1923. The German government, impressed by its security, acquired the rights to the machine and adapted it for military use. Throughout World War II, Germany and its allies encrypted military messages using these machines and, by 1945, over 40,000 such machines were in use. The Germans considered its code to be unbreakable but mathematicians in Poland, France and the UK developed advanced techniques that broke its code, helping to shorten the war. How many keys are on the keyboard of this machine?
IT was patented in 1918 by the German engineer Arthur Scherbius (1878-1929), and produced commercially from 1923. The German government, impressed by its security, acquired the rights to the machine and adapted it for military use. Throughout World War II, Germany and its allies encrypted military messages using these machines and, by 1945, over 40,000 such machines were in use. The Germans considered its code to be unbreakable but mathematicians in Poland, France and the UK developed advanced techniques that broke its code, helping to shorten the war. How many keys are on the keyboard of this machine? THESE objects are standard measures for volume, weight and length, made on the instruction of Queen Elizabeth I between 1588 and 1601. Elizabeth’s standards were sent to 60 cities so that – for the first time – all merchants could share the same definition of measurement units. The queen had recognized that a common set of measurement standards across the country was crucial to commercial expansion.
THESE objects are standard measures for volume, weight and length, made on the instruction of Queen Elizabeth I between 1588 and 1601. Elizabeth’s standards were sent to 60 cities so that – for the first time – all merchants could share the same definition of measurement units. The queen had recognized that a common set of measurement standards across the country was crucial to commercial expansion. IT was held at the Royal Mint, London, from 1818. IT housed weights collected by British consuls stationed overseas, each drawer containing standard weights from the place marked on the front. Each set of weights was then carefully compared with the British standard. The situation was complicated, though. Take Rome. If you were a merchant buying gold and silver you needed one set of weights. Medicines required another. Commercial goods involved a third. Dry goods such as salt had their own measures.
IT was held at the Royal Mint, London, from 1818. IT housed weights collected by British consuls stationed overseas, each drawer containing standard weights from the place marked on the front. Each set of weights was then carefully compared with the British standard. The situation was complicated, though. Take Rome. If you were a merchant buying gold and silver you needed one set of weights. Medicines required another. Commercial goods involved a third. Dry goods such as salt had their own measures. The miniature – a rather large miniature – is a dominant part of the Trade and Travel section. The model, still in perfect condition, was crafted by the Japanese shipbuilders, Ishikawajima Harima Heavy Industries of Kure, later known as IHI Corp. IT was one of a series of three built to pick up cargoes of crude oil from the Middle East Gulf for Japan. When completed in 1973 it was described as “the largest moving object ever built,” with a length of 378.85m.
The miniature – a rather large miniature – is a dominant part of the Trade and Travel section. The model, still in perfect condition, was crafted by the Japanese shipbuilders, Ishikawajima Harima Heavy Industries of Kure, later known as IHI Corp. IT was one of a series of three built to pick up cargoes of crude oil from the Middle East Gulf for Japan. When completed in 1973 it was described as “the largest moving object ever built,” with a length of 378.85m. IT is a model that represents and East Indiaman, a merchant ship carrying valuable goods thousand of miles across treacherous oceans. The 16th and 17th centuries saw an explosion of British trade with overseas nations. The East India company, founded in 1600, came to dominate Britain’s imports and exports. It was so successful it chartered a huge fleet of ships (known as East Indiamen) that could rival any army. The company eagerly took on new navigational techniques. One was known as lunar distances, first introduced in the 1760s. Can you locate the scientific instruments and tables of numbers that helped navigating officers to find their position?
IT is a model that represents and East Indiaman, a merchant ship carrying valuable goods thousand of miles across treacherous oceans. The 16th and 17th centuries saw an explosion of British trade with overseas nations. The East India company, founded in 1600, came to dominate Britain’s imports and exports. It was so successful it chartered a huge fleet of ships (known as East Indiamen) that could rival any army. The company eagerly took on new navigational techniques. One was known as lunar distances, first introduced in the 1760s. Can you locate the scientific instruments and tables of numbers that helped navigating officers to find their position? THIS book was written by the mathematician and natural philosopher Isaac Newton in the late 1660s, although it was not published until 1736. Newton wanted to create a mathematical language to describe physical quantities that are constantly changing. These situations can be described by differential equations, and occur widely in everything from bombs and astronomy to electricity and economics. Newton used the term ‘fluxions’ to describe this mathematics.
THIS book was written by the mathematician and natural philosopher Isaac Newton in the late 1660s, although it was not published until 1736. Newton wanted to create a mathematical language to describe physical quantities that are constantly changing. These situations can be described by differential equations, and occur widely in everything from bombs and astronomy to electricity and economics. Newton used the term ‘fluxions’ to describe this mathematics. This machine was built in 1935 to help Manchester University physicists solve problems in a variety of fields ranging from electrical power transmission to bomb production. During WWII, IT was put to use in military research. One secret project involved calculating the mathematics of uranium enrichment, vital in manufacturing the atomic bomb dropped in Hiroshima. What is the name of this machine?
This machine was built in 1935 to help Manchester University physicists solve problems in a variety of fields ranging from electrical power transmission to bomb production. During WWII, IT was put to use in military research. One secret project involved calculating the mathematics of uranium enrichment, vital in manufacturing the atomic bomb dropped in Hiroshima. What is the name of this machine? IT was built in 1981 at Brunel University in London, as an attempt to recreate the ‘neural networks’ of the brain. This early Artificial Intelligence machine worked, until 1995, on a variety of projects, from banknote recognition to voice analysis, and from fetal growth monitoring in hospitals to covert surveillance for the Home Office. Artificial neural networks learn form their surroundings using the mathematics of probability.
IT was built in 1981 at Brunel University in London, as an attempt to recreate the ‘neural networks’ of the brain. This early Artificial Intelligence machine worked, until 1995, on a variety of projects, from banknote recognition to voice analysis, and from fetal growth monitoring in hospitals to covert surveillance for the Home Office. Artificial neural networks learn form their surroundings using the mathematics of probability. IT is a piece from the London University Computing Service. IT was the most powerful computer in the world when released in 1962. IT was partly founded by BP, which used IT to calculate routes for its oil tanker fleet. The problem of finding the shortest route involved great complexity. There are millions of different routes one tanker can take to visit just a handful of different ports. Mathematical techniques developed during WWII made the problem manageable.
IT is a piece from the London University Computing Service. IT was the most powerful computer in the world when released in 1962. IT was partly founded by BP, which used IT to calculate routes for its oil tanker fleet. The problem of finding the shortest route involved great complexity. There are millions of different routes one tanker can take to visit just a handful of different ports. Mathematical techniques developed during WWII made the problem manageable. IT was invented and constructed by the Japanese ocean scientist Shizuo Ishiguro at the National Institute of Oceanography, Wormley, 1960-2007. In 1956, Ishiguro moved to the institute in Surrey, where he made THIS 6ft high electronic model. IT divided a body of water such as the North Sea into an electrical grid, with the flows of electricity designed to mirror those of the water. This modeling could help predict the strength, location and timing of storm surges.
IT was invented and constructed by the Japanese ocean scientist Shizuo Ishiguro at the National Institute of Oceanography, Wormley, 1960-2007. In 1956, Ishiguro moved to the institute in Surrey, where he made THIS 6ft high electronic model. IT divided a body of water such as the North Sea into an electrical grid, with the flows of electricity designed to mirror those of the water. This modeling could help predict the strength, location and timing of storm surges. IT was made around 1666 by Jamal al-Din at Lahore, Pakistan. IT is in essence a model of the universe that an astronomer could hold in their hands. Popular in Medieval and Renaissance Europe, ITs many uses included timekeeping, astrology and surveying. IT is a two-dimensional depiction of the heavens. IT has ancient origins and IT became a technological wonder in the hands of Islamic astronomers from where it spread to Europe.
IT was made around 1666 by Jamal al-Din at Lahore, Pakistan. IT is in essence a model of the universe that an astronomer could hold in their hands. Popular in Medieval and Renaissance Europe, ITs many uses included timekeeping, astrology and surveying. IT is a two-dimensional depiction of the heavens. IT has ancient origins and IT became a technological wonder in the hands of Islamic astronomers from where it spread to Europe. Without IT we cannot understand the heavens. ITs basic elements were drawn together in Ancient Greece in about 300BC. This work, which was the basis f0r over 2000 years of mathematics teaching, was first translated into English by Henry Billingsley in 1570. In the 19th century mathematicians began expanding IT to explore abstract concepts.
Without IT we cannot understand the heavens. ITs basic elements were drawn together in Ancient Greece in about 300BC. This work, which was the basis f0r over 2000 years of mathematics teaching, was first translated into English by Henry Billingsley in 1570. In the 19th century mathematicians began expanding IT to explore abstract concepts. THIS calculating machine was invented by Sir Samuel Morland and made by Henry Sutton and Samuel Knibb, London, 1664. IT was used to simplify calculations involving triangles. Surveyors rely extensively on this practice as it enables huge areas to be surveyed using the lengths and angles of triangles. The operator used THIS machine by ‘drawing’ the problem on IT. IT contained three rulers which could be moved using dials to make a triangle of required size. Angles and lengths could then be read off directly. However, it was expensive and did not catch on.
THIS calculating machine was invented by Sir Samuel Morland and made by Henry Sutton and Samuel Knibb, London, 1664. IT was used to simplify calculations involving triangles. Surveyors rely extensively on this practice as it enables huge areas to be surveyed using the lengths and angles of triangles. The operator used THIS machine by ‘drawing’ the problem on IT. IT contained three rulers which could be moved using dials to make a triangle of required size. Angles and lengths could then be read off directly. However, it was expensive and did not catch on. IT includes pioneering diagrams that Florence Nightingale developed to show, at a glance, the proportion of soldiers killed each month from disease. It was written in 1859 by Harriet Martineau with Florence Nightingale, the latter a mathematician and nursing reformer. Nightingale ran British military hospitals in the 1850s Crimean War and was horrified by how many soldiers died from preventable diseases.
IT includes pioneering diagrams that Florence Nightingale developed to show, at a glance, the proportion of soldiers killed each month from disease. It was written in 1859 by Harriet Martineau with Florence Nightingale, the latter a mathematician and nursing reformer. Nightingale ran British military hospitals in the 1850s Crimean War and was horrified by how many soldiers died from preventable diseases. IT was used in developing countries to monitor children’s growth. IT allows nurses to see at a glance whether children were heavier or lighter than average for their height. IT measures people against a statistical average and classifies them as normal or abnormal.
IT was used in developing countries to monitor children’s growth. IT allows nurses to see at a glance whether children were heavier or lighter than average for their height. IT measures people against a statistical average and classifies them as normal or abnormal.  IT is one of the three random-number-selector machines used in the UK’s National Lottery live draws held since 1994. One at a time, the numbers flashed up on millions of TV screens. Somebody was about to become a millionaire. Yet for all its modern glitz, IT performed and ancient and venerated statistical task – the selection of a random number.
IT is one of the three random-number-selector machines used in the UK’s National Lottery live draws held since 1994. One at a time, the numbers flashed up on millions of TV screens. Somebody was about to become a millionaire. Yet for all its modern glitz, IT performed and ancient and venerated statistical task – the selection of a random number.  THIS machine modelled the British economy using the flow of water to represent money. IT was made for the London School of Economics in 1952 by Bill Phillips, an engineer turned economist. Economists such as Phillips used mathematical equations to describe economic behavior and THIS machine solved them in a visual way. Approximately fourteen machines were built, and THIS particular machine was used as a teaching aid at the London School of Economics. IT ran until May 1992. What is the relationship between the income you have and the tax you pay and how is it modelled by this machine?
THIS machine modelled the British economy using the flow of water to represent money. IT was made for the London School of Economics in 1952 by Bill Phillips, an engineer turned economist. Economists such as Phillips used mathematical equations to describe economic behavior and THIS machine solved them in a visual way. Approximately fourteen machines were built, and THIS particular machine was used as a teaching aid at the London School of Economics. IT ran until May 1992. What is the relationship between the income you have and the tax you pay and how is it modelled by this machine?
Do you know that the gallery design is driven by airflow equations used in aviation? The layout and lines for the gallery represent the air that would have flowed around this historic aircraft in flight, from the positioning of the showcases and benches to the 3-dimensional curved surfaces of the central pod structure.
Can you estimate how tall this tower is?
Do you think it is the tallest building in London?
If you were to pack IT in a box, what kind of box would you choose and what dimensions would that box have? If you wanted to make a miniature version of this chair what ratio would you use?
How do you think this machine works?
Can you estimate the volume, weight and length of these standard measures?
How could the merchants have compared the weights of all these products?
Do you know that IT was powered by 45,000hp steam turbines to a single shaft, and was said to have a turning circle shorter than three times its length? What shape of a ship hull produces less resistance at high speeds and thus keeps fuel costs down?
What was the greatest scientific and commercial problem of the 17th and 18th centuries that caused devastating navigation errors?
What is the name of the mathematics describing physical quantities that are constantly changing?
What would you do if you wanted to make an artificial brain?
What piece from the London University computer is displayed in the gallery?
Can you find an earlier machine that attempted to provide accurate surge and tidal prediction?
What mathematical technique was used to achieve the two-dimensional depiction of the heavens on this instrument?
Who was the Greek mathematician who wrote the elements of this mathematical branch?
What is the name of the mathematics that studies the relationships between side lengths and angles of triangles and the applications of these relationship?
What was Florence Nightingale’s primary mathematical interest?
Have you seen this kind of chart before?
What is the mathematics involved in lottery drawings?
ANSWERS
- Handley Page H.P. 39 “Gugnunc” Aeroplane
- Model of the National Westminster Tower (now Tower 42)
 LE CORBUSIER CHAIR, 1930 – Form and Beauty
LE CORBUSIER CHAIR, 1930 – Form and Beauty- Enigma Machine, 1934
- Elizabethan Weights and Measures – Trade and Travel
- Cabinet of Foreign Weights, 1919-20 – Trade and Travel
- Globtik Tokyo steams into Science Museum’s new Maths Gallery
- Instruments for Lunar-Distance Navigation – Trade and Travel
- The Method of Fluxions and Infinite Series, by Isaac Newton, 1736
- Differential Analyser, 1935
- Wisard Pattern-Recognition Machine, 1981
- Ferranti Atlas computer
- Electronic Ocean Model
- Islamic astrolabe
- Geometry
- Trigonometry Instrument
- England and Her Soldiers, by Harriet Martineau, 1859
- Height and Weight Chart, 1992
- Guinevere, Random Number Selector
- Phillip’s Economic Computer
Tower 42 is the third-tallest skyscraper in the City of London and the eighth-tallest in Greater London. It was the tallest in the United Kingdom from 1980 to 1991.
See dimensions to check your answer!
Check out the collection “Le miniature” for inspiration!

There are 26 keys on the keyboard, each representing a letter of the alphabet. There are no numerals, no keys for punctuation or spaces, and no upper or lower case. You can learn more about how this machine works on this online enigma machine simulator.
Avoirdupois weight (standard)
Exchequer standard pint measure of Queen Elizabeth I, 1601
Weights and original correspondence from the Castlereagh Collection for Tunis
After all the weights had been compared, merchants could finally refer to accurate conversion rates for each product.
Experiments by mathematicians and engineers in the 1860s had shown that a bulbous bow produced less resistance at high speeds than the sharper bows favored for large ships at the time.
The problem was finding the longitude (the ship’s east–west position).
“Nothing is so much wanted and desired at sea as the discovery of the longitude … In the judgment of able mathematicians and navigators, several methods have already been discovered, true in theory, though very difficult in practice”
— Longitude Act, 1714
Mathematical Treasure: Newton’s Method of Fluxions – Open Library
Today the mathematics that studies continuously changing quantities (Newton’s ‘fluxions’) is known as differential calculus. Calculus is a vital mathematical tool.
The Manchester Differential Analyzers
Playing by the rules – People and machines have much in common
IT is the console from the University of London’s machine.
Modelling the oceans | Understanding storm surges in the North Sea: Ishiguro’s electronic modelling machine . pdf
The AIR Coastal Flood Model for Great Britain – AIR Worldwide
Lord Kelvin’s (William Thomson) First Tide Predicting Machine, 1876
Stereographic projection.
Elements of Geometry, by Euclid, first English translation, 1570
HELLENISTIC MATHEMATICS – EUCLID
Euclid
Examples for Trigonometry
Trigonometry is the study of the relationships between side lengths and angles of triangles and the applications of these relationships.
Florence Nightingale: the pioneer statistician
Florence Nightingale’s primary mathematical interest was statistics. She would call it ‘the most important science in the world’. Nightingale was elected the first female member of the Statistical Society (now the Royal Statistical Society) in 1858, just two years after returning from the Crimea.
Growth Charts are a standard part of any checkup, and they show health care providers how kids are growing compared with other kids of the same age and gender.
Lottery mathematics
Probability
How does the economy work?
The more income you have the more tax you pay. Moniac is designed so that the fuller the income tank, the faster water flows out at the top as tax.
Mathematics : How it Shaped Our World
MATHEMATICS: THE WINTON GALLERY
- COLLECTION | GALLERY GUIDE . pdf
- Memories of the Ferranti Atlas computer
- Handley Page H.P. 39 “Gugnunc” Aeroplane
- Model of Richard Seifert’s NatWest Tower.1:250 scale
- Phillip’s Economic Computer
- The UCLA Differential Analyzer: General Electric in 1947
- SCHOOL INFO
- STORIES ABOUT MATHEMATICS
- MATHEMATICS: THE WINTON GALLERY AT LONDON’S SCIENCE MUSEUM BY ZAHA HADID ARCHITECTS
- Maths gallery at the UK’s Science Museum takes flight
- Science Museum’s maths gallery soars with stunning Zaha Hadid design
- Zaha Hadid Architects: The Winton Gallery opens at the Science Museum
The Atlas Story . pdf
The Story of ATLAS – A computer . pdf
GLAMi Nomination: Handley Page VR
HANDLEY PAGE VR – Revealing the magic of mathematics through the Science Museum’s first interactive VR experience.
Aviation, Computers, Economics, Navigation, Oceans, Statistics
Mathematics: The Winton Gallery at the Science Museum, London
Zaha Hadid Team Turns Airflow into Architecture
TO BE CONTINUED…
- Bletchley Park – Self guided resources
- History of the Enigma – Crypto Museum | Scherbius’ Enigma
- Nautical Almanac – The Royal Observatory Greenwich
- Tabulating the Heavens: Computing the Nautical Almanac in 18th-Century England
- The Manchester Differential Analyzers
HOW ALAN TURING CRACKED THE ENIGMA CODE
How did the Enigma machine work? | How Enigma Machines Work
How the Enigma Works

